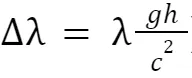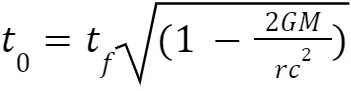Works Referenced
1 Hubble, Edwin P. The Observational Approach to Cosmology. Oxford University Press, 1937.
2 Einstein, Albert. “Cosmological Considerations in the General Theory of Relativity.” Annalen der Physik, vol. 354, no. 7, 1917, pp. 769–822.
3 Hubble, Edwin P. “NGC 6822, a Remote Stellar System.” The Astrophysical Journal, vol. 62, 1925, pp. 409–433.
4 Kragh, Helge. “Albert Einstein’s Finite Universe.” Masters of the Universe: Conversations with Cosmologists of the Past, Oxford, 2014; online edn, Oxford Academic, 19 Mar. 2015. Accessed 4 Mar. 2024. doi:10.1093/acprof:oso/9780198722892.003.0005.
5 Nussbaumer, Harry. “European Physics Journal — History.” European Physics Journal — History, vol. 39, 2014, pp. 37–62. doi:10.1140/epjh/e2013–40037–6.
6 Heisenberg, Werner. Encounters with Einstein : And Other Essays on People, Places, and Particles. Princeton University Press, 1989.
7 Wheeler, John Archibald, and Kenneth Wilson Ford. Geons, Black Holes, and Quantum Foam: A Life in Physics. W. W. Norton & Company, 2010.
8 Peebles, P. J. E. Principles of Physical Cosmology. Princeton University Press, 1993.
9 Halpern, Paul. “How Richard Feynman Convinced The Naysayers 60 Years Ago That Gravitational Waves Are Real.” Forbes, 7 Mar. 2017, www.forbes.com/sites/startswithabang/2017/03/07/how-richard-feynman-convinced-the-naysayers-that-gravitational-waves-were-real-60-years-ago/?sh=1b15b353ab11. Accessed 4 Mar. 2024.
10 Romano, Joseph D., and Neil J. Cornish. “Detection Methods for Stochastic Gravitational-Wave Backgrounds: A Unified Treatment.” Living Reviews in Relativity, vol. 20, no. 1, 2017, p. 2. doi:10.1007/s41114–017–0004–1.
11Adler, Ronald J., Brendan Casey, and Ovid C. Jacob. “Vacuum catastrophe: An elementary exposition of the cosmological constant problem.” American Journal of Physics, vol. 63, no. 7, 1995, pp. 620–626. doi:10.1119/1.17850.
12 Planck Collaboration. “Planck 2018 Results: VI. Cosmological Parameters.” Astronomy & Astrophysics, vol. 641, 2020, p. A6. Crossref, https://doi.org/10.1051/0004-6361/201833910.
13 Sandved, Patrik Ervin. “A Possible Explanation of the Redshift.” Journal of the Washington Academy of Sciences, vol. 52, no. 2, 1962, pp. 31–35.
14 Marmet, Paul. “A Possibility of Gravitational Redshifts.” Canadian Journal of Physics, vol. 41, no. 1, 1963, pp. 147–152.
15 Assis, André K.T. “A Steady-State Cosmological Model Derived Relativistically.” Progress in Physics, vol. 3, no. 3, July 2007, pp. 88–92.
16 Gentry, Robert V. “A New Redshift Interpretation.” Modern Physics Letters A, vol. 12, no. 37, Dec. 1997, pp. 2919–25. doi:10.1142/s0217732397003034.
17 Bunn, Edward F., and David W. Hogg. “The Kinematic Origin of the Cosmological Redshift.” American Journal of Physics, vol. 77, no. 8, 2009, pp. 688–694.
18 Einstein, Albert. “Cosmological Considerations in the General Theory of Relativity.” Sitzungsberichte der Königlich Preussischen Akademie der Wissenschaften (Berlin),Part 1, 1917, pp. 142–152.